Is There A Winning Strategy To Roulette

4/9/20224/9/2022

santetupfisuta.netlify.com › 〓〓〓 Is There A Winning Strategy To Roulette 〓〓〓

Is There A Winning Strategy To Roulette Average ratng: 7,6/10 6483 reviews

If there is someone on the casino or anywhere in the online world telling you that they can tell you a surefire strategy to win the game, they are lying. A few strategies that can work have already been stated above. There is no guaranty of winning the roulette game because that would kill the purpose of the game in the first place. A roulette strategy has one aim and one aim only: to win. Most roulette strategies are based around telling players how they should bet and when to bet it. The game of roulette has more potential strategies than any other casino game.

Published Tuesday, Oct. 27, 2020, 1:34 pm

Join AFP's 100,000+ followers on Facebook

Purchase a subscription to AFP Subscribe to AFP podcasts on iTunes

News, press releases, letters to the editor: augustafreepress2@gmail.com

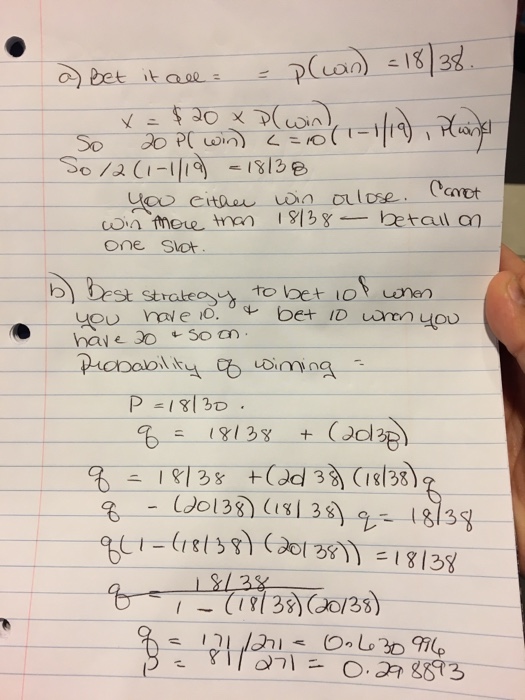
Advertising inquiries: freepress@ntelos.net

Many books and articles have been written on how to win the roulette. There are some great strategies out there, but in order to win, you have to either play on an Online Live Casino or prefer a European roulette. However, the most important thing is to choose the best strategy for your wallet.

Play at a live casino

There are two types of online casinos the RNG Casino, where all games are played automatically without a dealer, and the Live Casino that you can play with a real dealer. When you play RNG, the results are random, and there is no one to throw the ball or draw a card. Everything happens with a click, so you can’t follow any strategy. If you want to make money, then you should play on a Live casino NetBet.

European roulette

The European roulette has more advantages for the player than the American one. The main difference is that the American one has the double zero (00), which automatically increases the casino advantage to 5.26%, compared to the 2.70% of the European.

The best strategy for you

There are many strategies for all kinds of budgets. What you must do first is to decide the amount you want to spend. If you keep spending and then rethinking and re-planning, there is a chance you may hurt your finances.

Beginners

Is there a winning strategy to roulette numbers

First of all, choose the online casino that has many live roulette tables. Be patient and observe when one of them will create a series, for example, eight blacks in a row. If that happens, then bet on the opposite (i.e., red), and your bet will be doubled each time a red comes. It’s quite rare to get ten or more consecutive blacks in a row.

Martingale strategy

The Martingale strategy is the most played roulette strategy. Usually, winning is all about luck, but Martingale is quite effective, and players can use it to win. The player is required to double his bet after each loss until he reaches the first win that will return the losses and bring him a profit equal to his original bet. This can lead to incredibly high stakes if you lose in a few consecutive rounds, so you need to be prepared to stop when the stakes start to get too high for you.

Roulette strategy with a 64% chance of winning

This strategy can work only in the European Roulette. You must bet equally on two out of the three dozens of the roulette. The chance to win is 64%, which is high, considering that it’s a game based on luck. If you lose, you’ll need to stick to the following progress:

1 $ – 1 $
3 $ – 3 $
9 $ – 9 $
27 $ – 27 $

The chance of losing four consecutive spins is minimal, and that is why the 64% strategy is very effective.

The John Wayne strategy

This is a very useful and effective strategy that doesn’t need a lot of money. All you have to do is choose two random numbers from the middle column (e.g., 14 and 29), and you will bet € 1 on them. Also, bet € 1 in their corners, as shown in the image below. As you can see, we have bet only € 10, and we have covered 18 numbers (that is, half of the roulette).

There are three different results:

Come 14 or 29 (according to our example). In this case, you win $72.
Let any of: 11, 13, 15, 17, 26, 28, 30, or 32 come. In this case, you win $18.
Let any of: 10, 12, 16, 18, 25, 27, 31, or 33 come. In this case, you win $1.

As you can see, the main idea is to have two bets on two prime numbers (14, 29) and the rest of the stakes to act as security. Losing $1 does not compare to the chance of winning $18 or $72. It’s a very simple strategy that we suggest you play with $100 initial capital to cope if you are incredibly unlucky.

Why cannot you succeed in roulette via using strategies?

Let's consider all possible common bets in roulette as the mathematical proof of the impossibility to win in roulette and determine their ME (Mathematical expectation) result.

In the general case, I of any bet in roulette can be calculated using the classical formula (1):

, (1)

Where is xi– event I,
Pi– The probability of the event I,
К – The total number of events forming a complete group.

The mathematical expectation of the European version:

The formula (1) for ME of any bets on 'European roulette' can be converted taking into the full probability, not collaborative events, that is Pwin.+ Ploss.=1, to the view with the game for N sectors (rooms), is equal to:
... (2)

Since the probability of winning Pwin in 'European roulette', while you are playing for N sectors (rooms), is equal to:

, that finally, we obtain the expression for ME for ANY bet in 'European roulette', when you are playing for N sectors (rooms), is equal to:... (3)
Let's calculate ME for each 'simple' (basic) bet in 'European roulette' (a wheel with one zero). The result of ME calculation for average basic bets is presented in table 1.

The mathematical expectation for the bets:

Table 1. Calculation of ME for 'simple' bets.

№	«Simple» bet	Winning		Loss	Calculation МО, у.е.
№	«Simple» bet	Payout	Probability	Probability	Calculation МО, у.е.
1.	Straight-Up	35:1	1/37	36/37	=35×1/37-36/37= -1/37
2.	Split	17:1	2/37	35/37	=17×2/37-35/37= -1/37
3.	Street	11:1	3/37	34/37	=11×3/37-34/37= -1/37
4.	Corner	8:1	4/37	33/37	=8×4/37-33/37= -1/37
5.	Six Line	5:1	6/37	31/37	=5×6/37-31/37= -1/37
6.	Column & Dozens	2:1	12/37	25/37	=2×12/37-25/37= -1/37
7.	Even Chance	1:1	18/37	19/37	=1×18/37-19/37= -1/37

As can we see from the table – ME is exactly equal to the value obtained by the formula (3). Let's sum up the results.

The player always loses even when he wins

If the gambler is playing at 'European roulette', (no matter where and how much he puts), he ALWAYS loses 1/37 part of the bet (bets). At the same ME of the game, it is not dependent on the outcome of the spin; that is, the player loses even when he wins. Or in other words, the player ALWAYS loses when he makes a bet at 'European Roulette' regardless of the outcome of the current spin.

Using the strategies of the bets:

For the mathematical proof of the winning's impossibility at 'European roulette' or 'American Roulette,' it is enough to expand any betting strategy on the 'basic' bet. As ME result of all bets is negative and equals -1/37 the size of the stake, then the total expectation of the game result will be negative and equal -1/37. The sum of all bets made by the player or -1/37 from the value of the average stake multiplied by the number of spins played by a gambler.

Assessing the ME of any strategy is enough to determine the value of the average bet while you are playing according to this strategy and taking into consideration all the rules of the transition from bet to bet, and multiplying the result by -1/37. The value of the average bet and sum of all of the bets are positive values; therefore ME is always less than zero, that is ME£0 and less than ME£-1/37 if used the progression because the average rate is bigger than 1.

Dispersion

Let's calculate the variance for any bet in 'European roulette', depending on how many sectors N (rooms) puts the gambler. Use the variance to determine the optimal Bank Kelly criterion for playing 'European roulette'.

Information

Bank on Kelly's criterion shows what should be the gambler's bank to the total balance of all games aspired to infinity.

In the general case, the variance of the player who is playing at the 'European roulette' in N sectors (rooms) can be calculated by the expression:

. (4)

The formula (4) for the variance D of any bets on the 'European roulette' can be converted taking into the full probability, not joint events, that is Pwin.+ Ploss.=1, to the mind:

. (5)

Is There A Winning Strategy To Roulette Numbers

Since the probability of winning Pwin in the 'European Roulette' while you are playing for N sectors (rooms) is equal to:

, finally, we get the expression for the dispersion D of any bet at the 'European roulette' when you are playing for N sectors (rooms), is:. (6)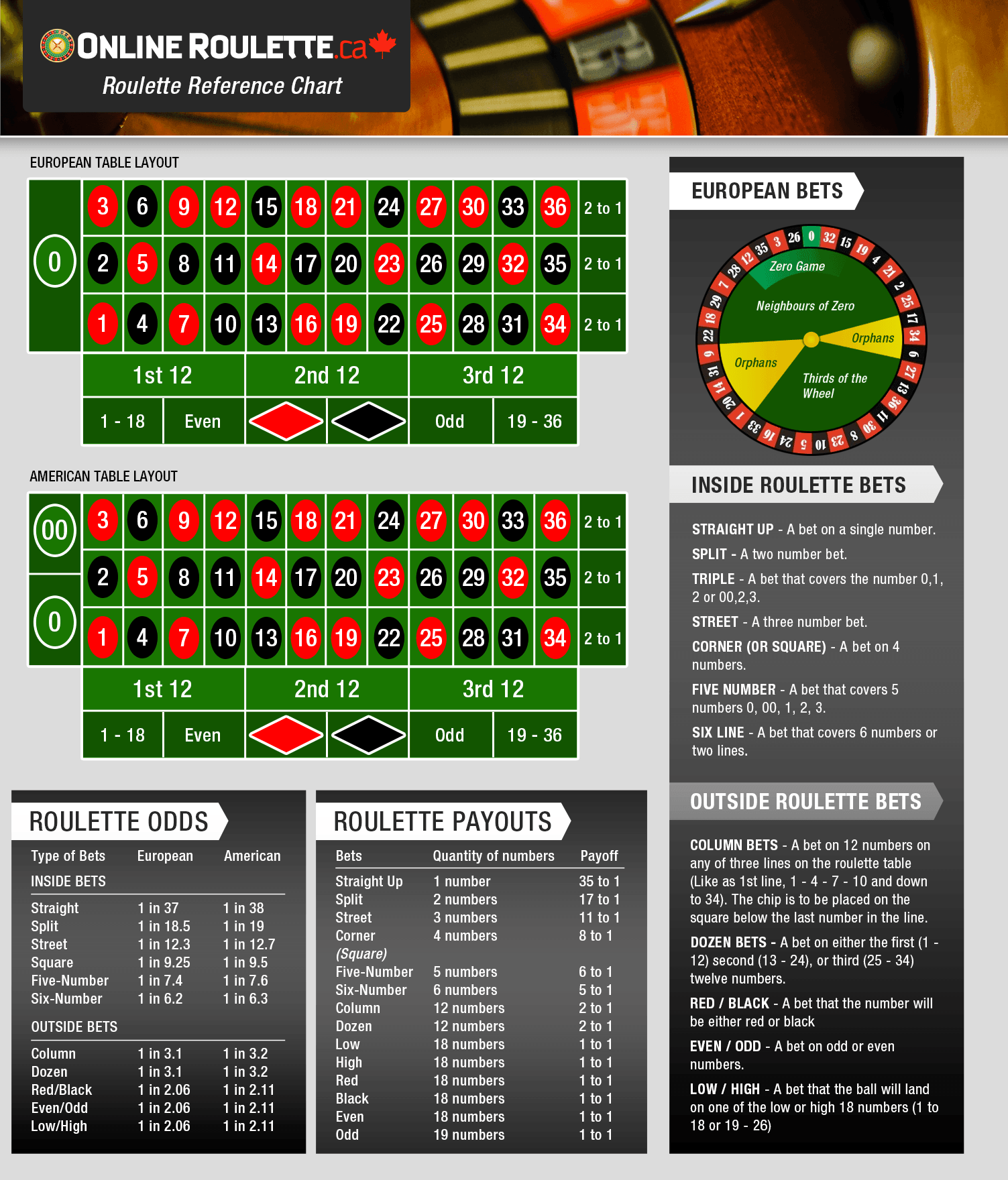 There

The magnitude of dispersion D has a positive value throughout the range of games in N sectors. That is an important detail.

Where it is possible to calculate the required bank for the game at 'European roulette' using Kelly's criterion:

. (7)

Using the expressions (3) and (6), we finally obtain the expression:

(8).

The formula (8) shows that the optimal amount of the bank, according to Kelly's criterion for playing at 'European Roulette' is a negative value.

Conclusion:

If the optimal value of the gambler's bank following Kelly's criterion is negative, then playing at 'European roulette' is generally not necessary, since the overall result of the balance of all players` games approach to zero, or the player has to lose all his money during the long game.

To assess the 'attractiveness' of the gambling, you can use generalised criteria, which is obtained as the ratio of required the gambler's bank following Kelly's criterion to the mathematical expectation of the game result that is:

(9).

This expression (9) can be understood that the smaller size of the gambler's bank at the mathematical expectation, the 'better' will be a game for the player. This optimal criterion can be interpreted as the criteria of minimal specific player's bank per unit of profit from the game.

The optimal criteria of the Кoptima game can only be used for evaluation of games with a positive expectation! If the game has a negative MT, it will not attract the attention of the gamblers. For 'European roulette' MT -1/37, that is less than zero, so 'European roulette' as the game is not 'attractive' for gamblers. That is why it is impossible to win in roulette, regardless of which betting strategies and tactics selection of items you use.

Note: the formula (3), (5) and (8) can be obtained for 'American roulette' with two sectors of zero: 0 and 00.

Similar materials

Where to play?

Is There A Good Strategy For Roulette

Choosing an honest is an important step before you start playing in live games or slots. Our highly recommended is Fastpay casino. They will send the money within 5 minutes.

Is There A Winning Strategy For Roulette

Add comment

santetupfisuta.netlify.com